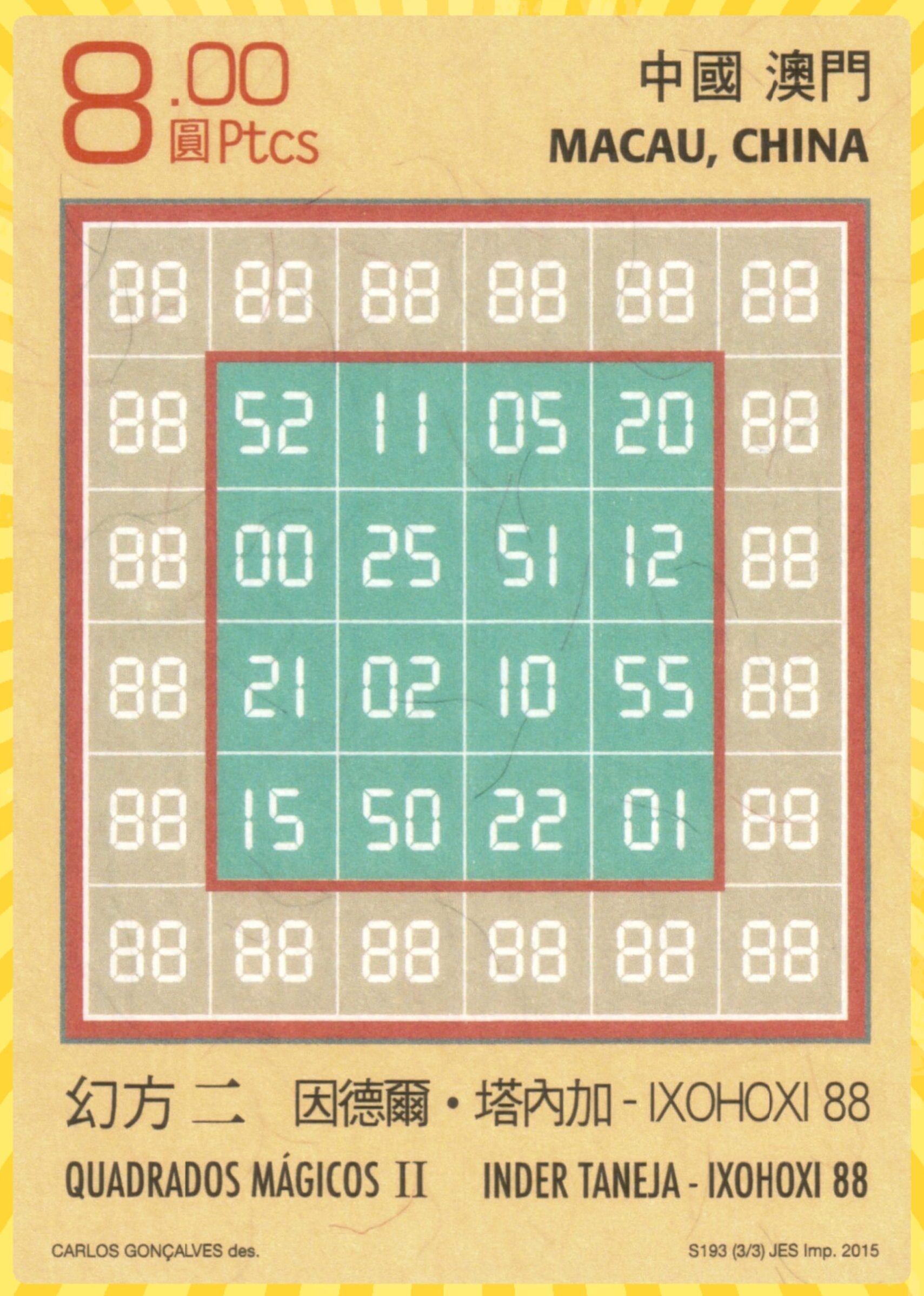
Recently, author worked with cornered Magic Squares based on two-by-two divisions. These works can be accessed at the following links:
1. Inder Jeet Taneja, Cornered Magic Squares of Orders 5 to 10
2. Inder Jeet Taneja, Cornered Magic Squares of Orders 11 to 13
3. Inder Jeet Taneja, Cornered Magic Squares of Orders 14 to 24
Also, the author worked on even order double digits bordered magic squares. This works starts from order 8 and goes up to order 40. See below the links:
The work for order of type 4k, k=1, 2, 3, … i.e., for orders 8, 12, 16, 20, 24, 28, 32, 36 and 40:
1. Two Digits Bordered Magic Squares Multiples of 4: Orders 8 to 24.
2. Two Digits Bordered Magic Squares of Orders 28 and 32.
3. Two digits Bordered Magic Squares of Order 36.
4. Two digits Bordered Magic Squares of Order 40.
The work is for the order of type 4k+2, k=1, 2, 3, …, i.e., for the orders 10, 14, 18, 22, 26, 30, 34 and 38:
1. Two digits Bordered Magic Squares of Orders 10, 14, 18 and 22.
2. Two digits Bordered Magic Squares of Orders 26 and 30.
3. Two digits Bordered Magic Squares of Order 34.
4. Two digits Bordered Magic Squares of Order 38.
This work is for magic square of order 23. It includes four type of magic squares:
1. Traditional centered magic squares.
2. Bordered magic squares with magic squares of order 3.
3. Double digits bordered magic squares.
4. Cornered magic squares.
Double digits and cornered magic squares can also be constructed using software, the reader can follow H. White’s site: Magic Squares. Also, the cornered magic squares can be rotated in all the four directions again by using software from H. White’s site.
For the previous work on odd orders from 3 to 19 see the following links:
1. Inder J. Taneja, Odd Order Magic Squares: Orders 3 to 15.
2. Inder J. Taneja, Magic Squares of Orders 17 and 19.
3. Inder J. Taneja, Magic Squares of Order 21.
To have better idea, pdf file of total 370 magic squares of order 23 is attached for download.
Magic Squares of Order 23
As explained above, the construction of magic squares of order 23 are of four types. Below are examples of each case. In case of second type there are two possibilities. One centered border of order 3. Second, cornered border of order 3.
1. Traditional Centered Magic Squares

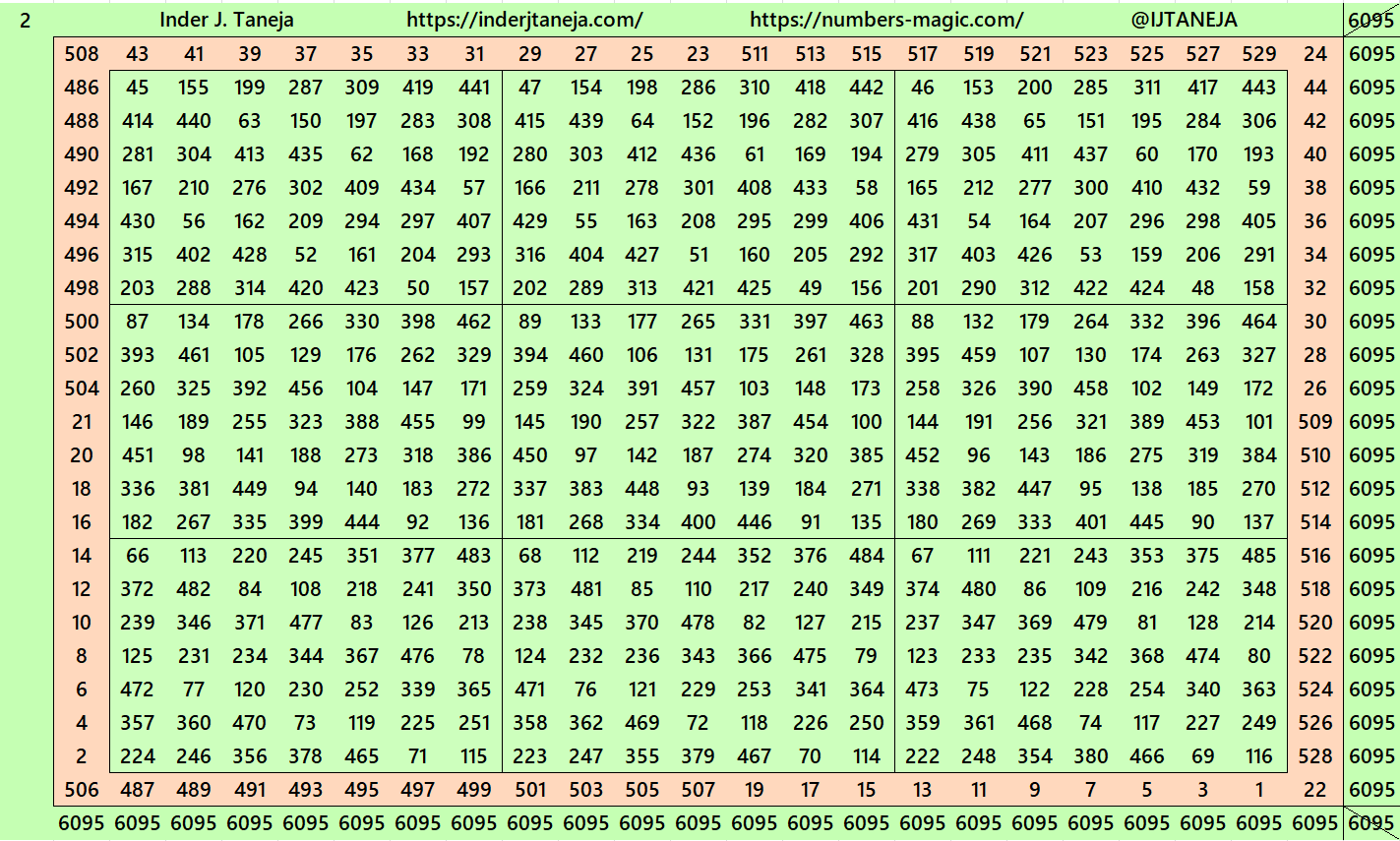
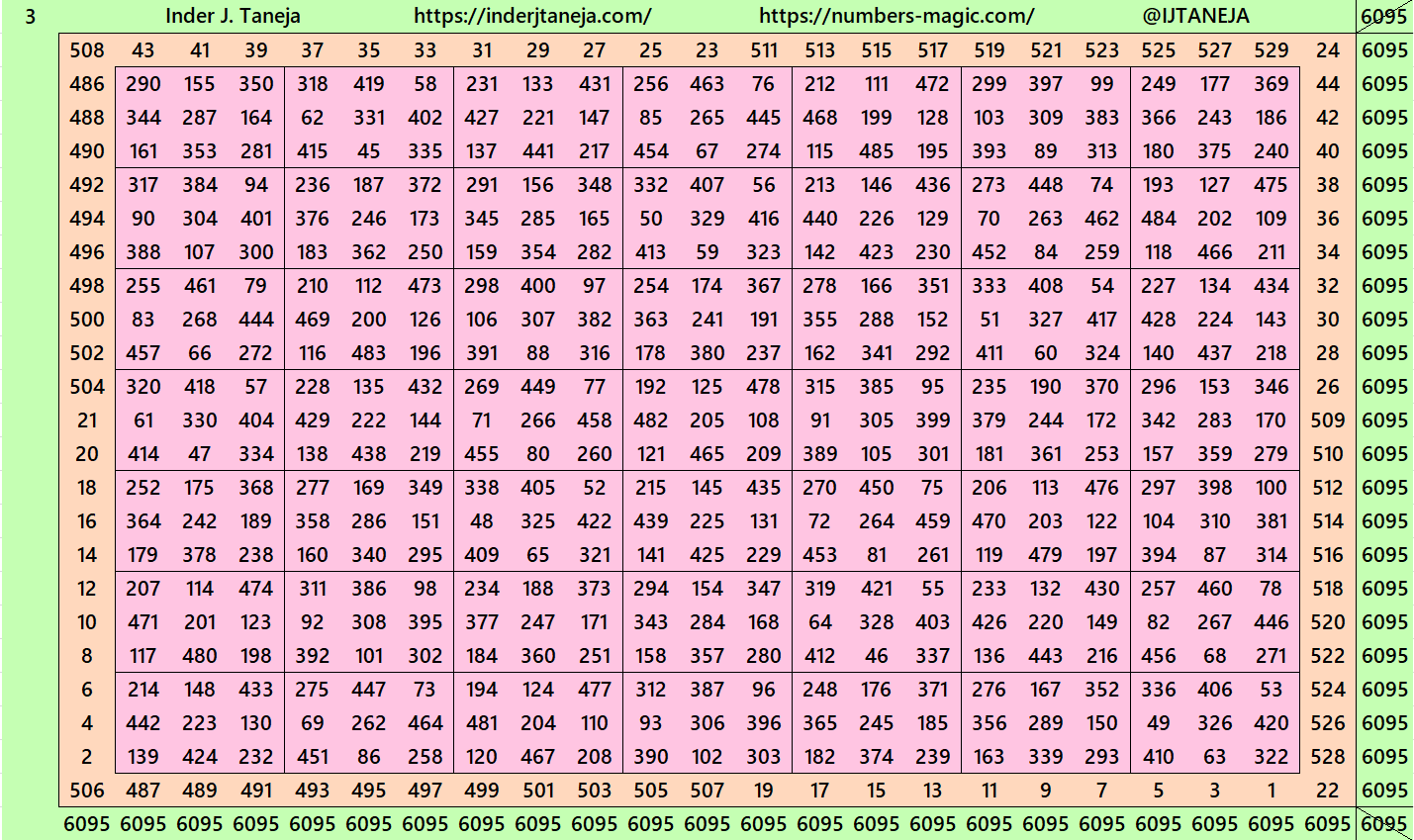

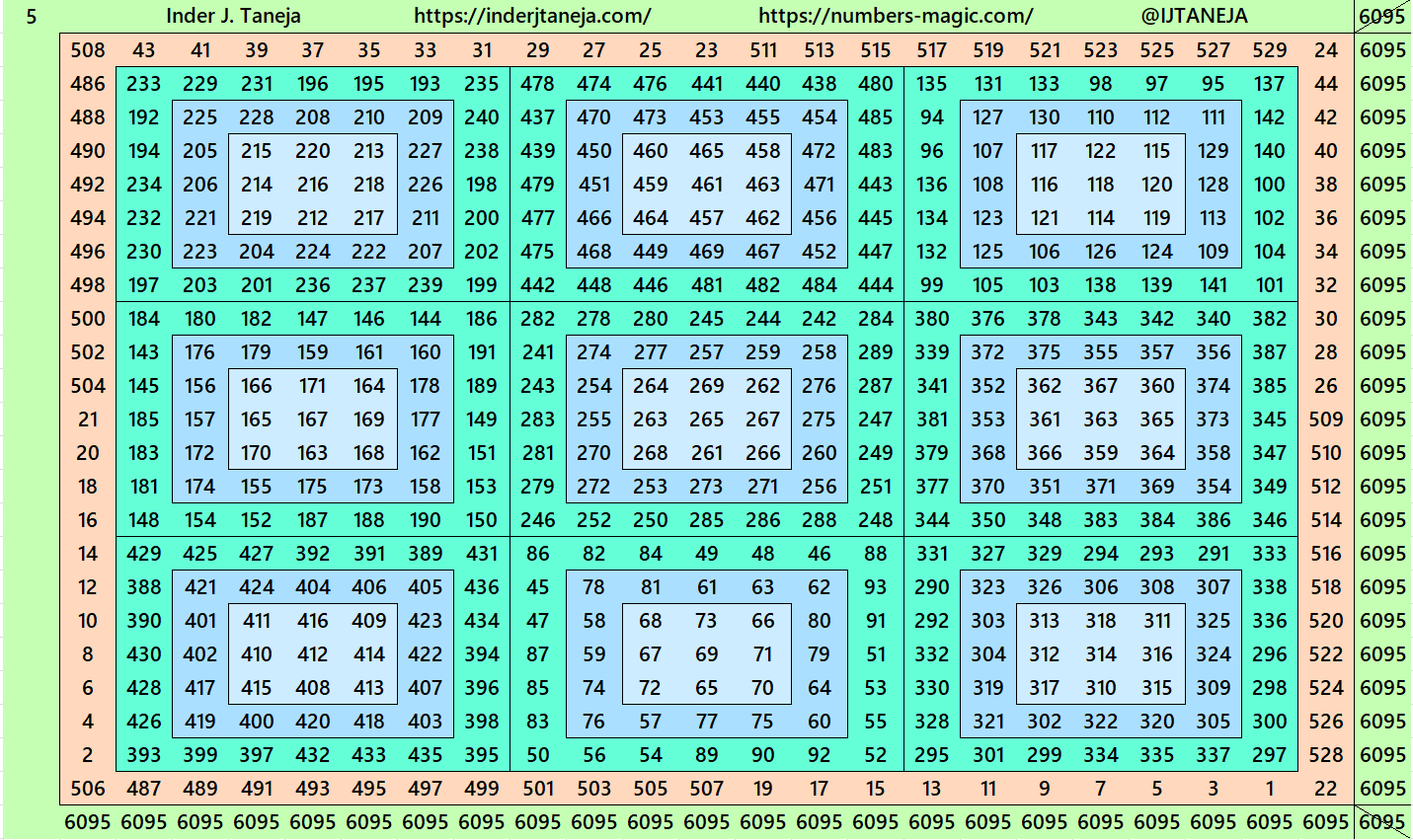
2. Part 1: Bordered Magic Squares of Order 3

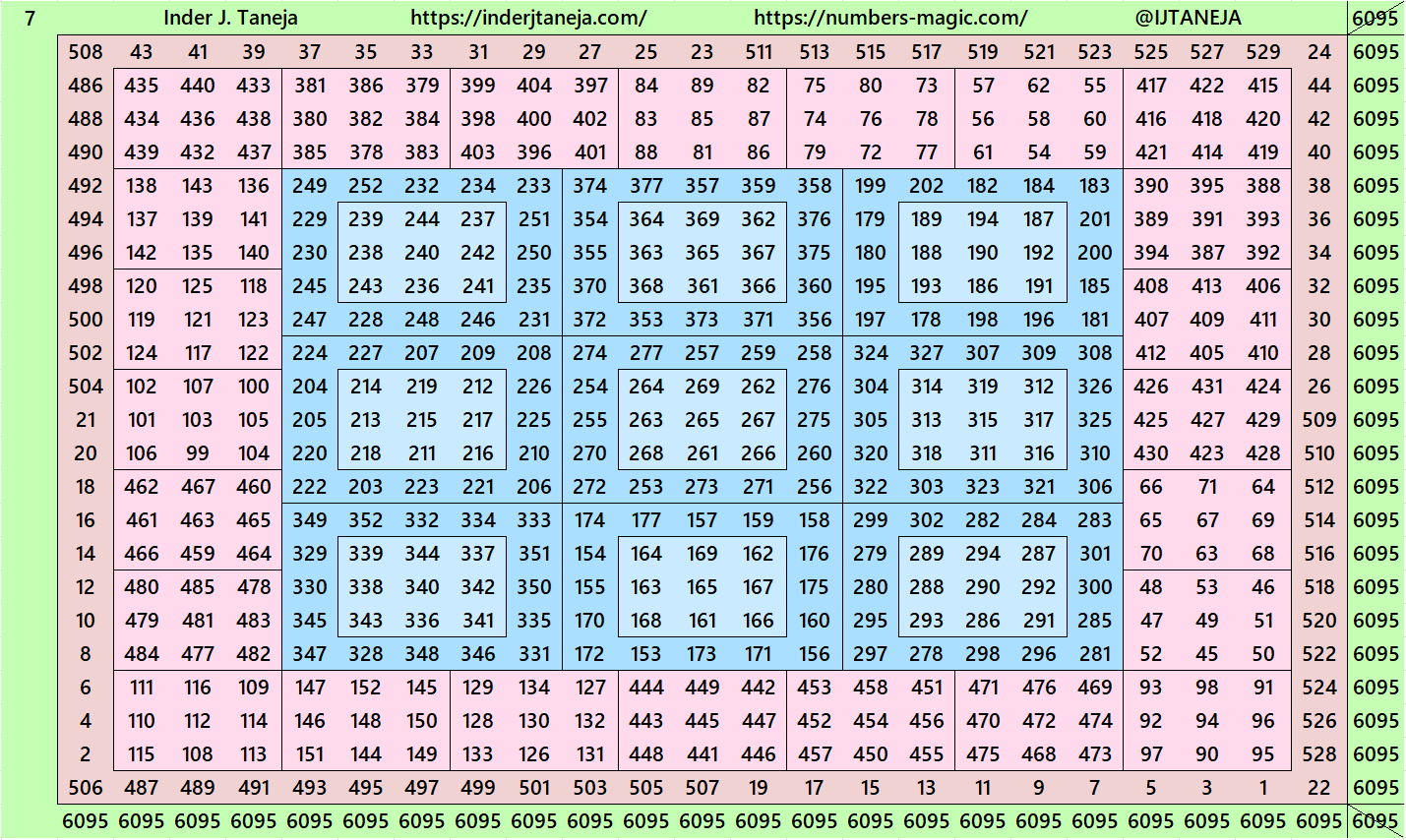
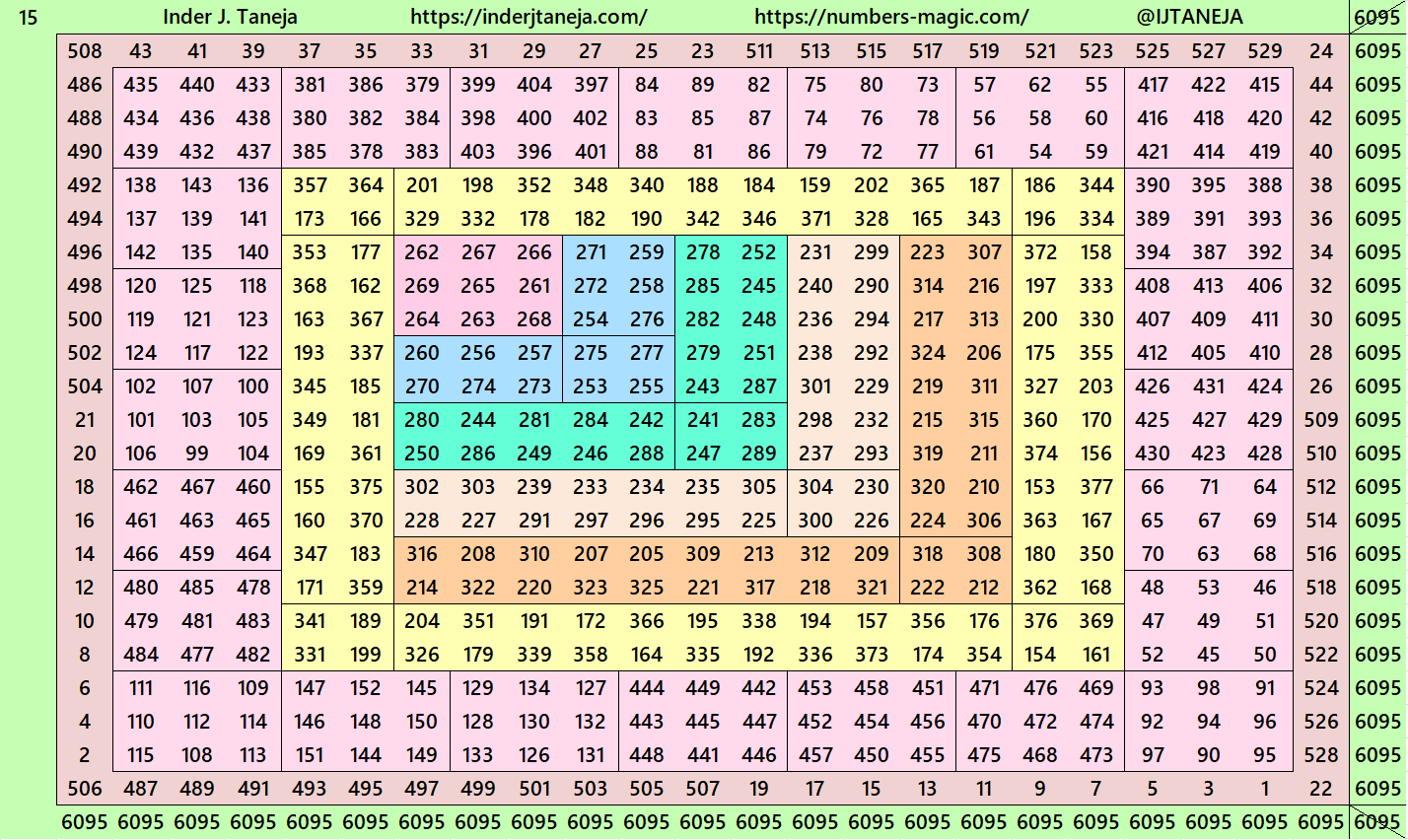
2. Part 2: Cornered with Magic Squares of Order 3

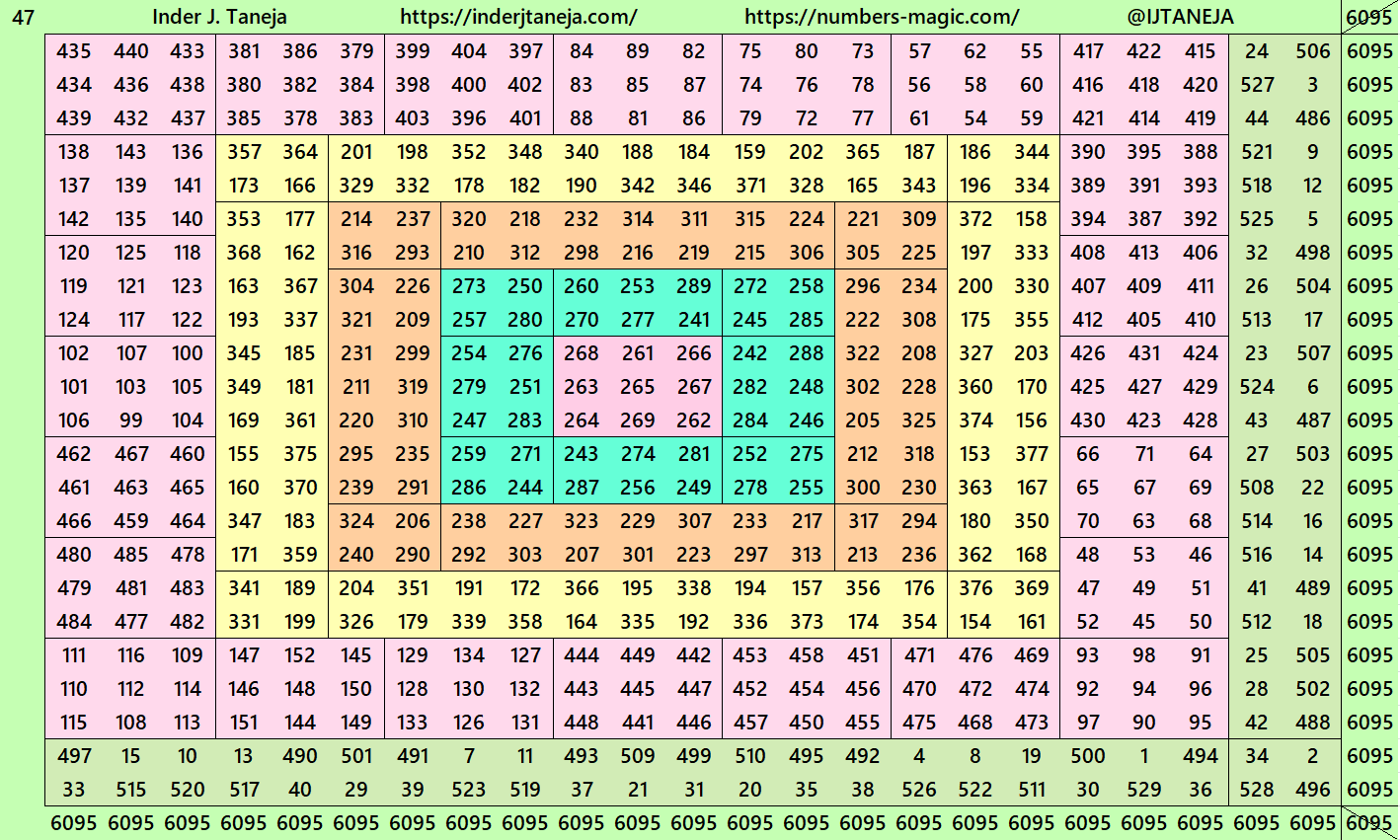

3. Double Digits Magic Squares
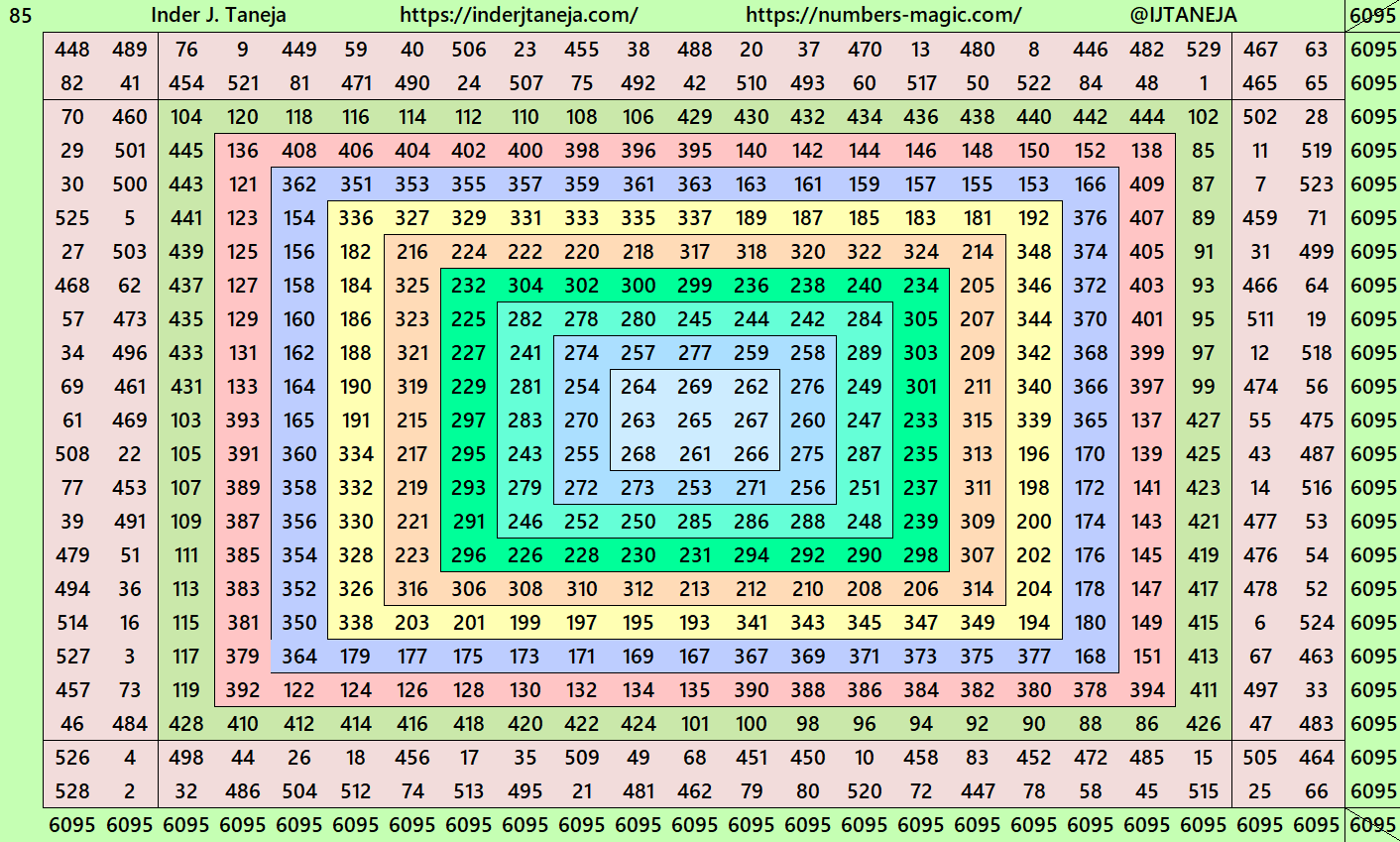
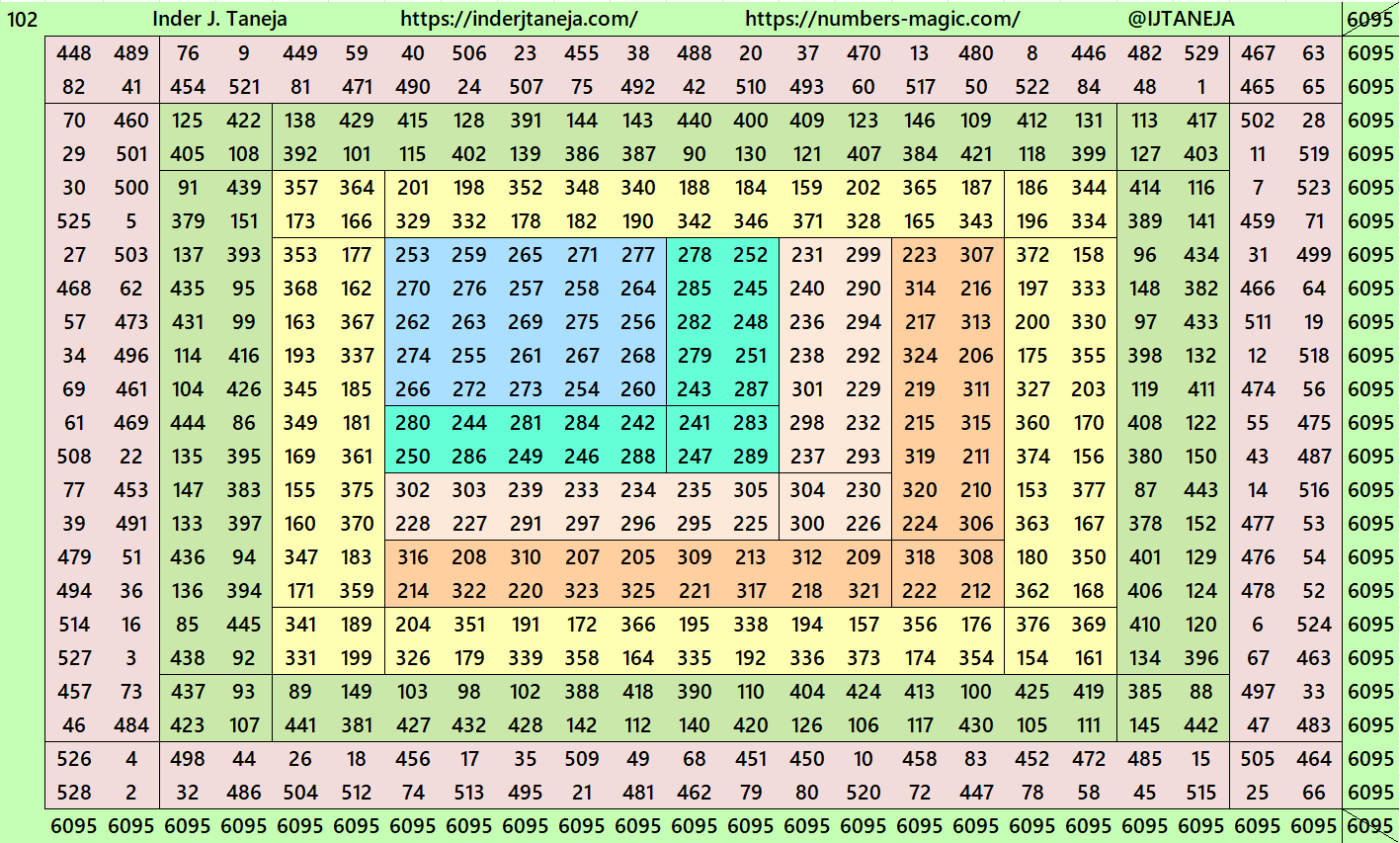

4. Cornered Magic Squares
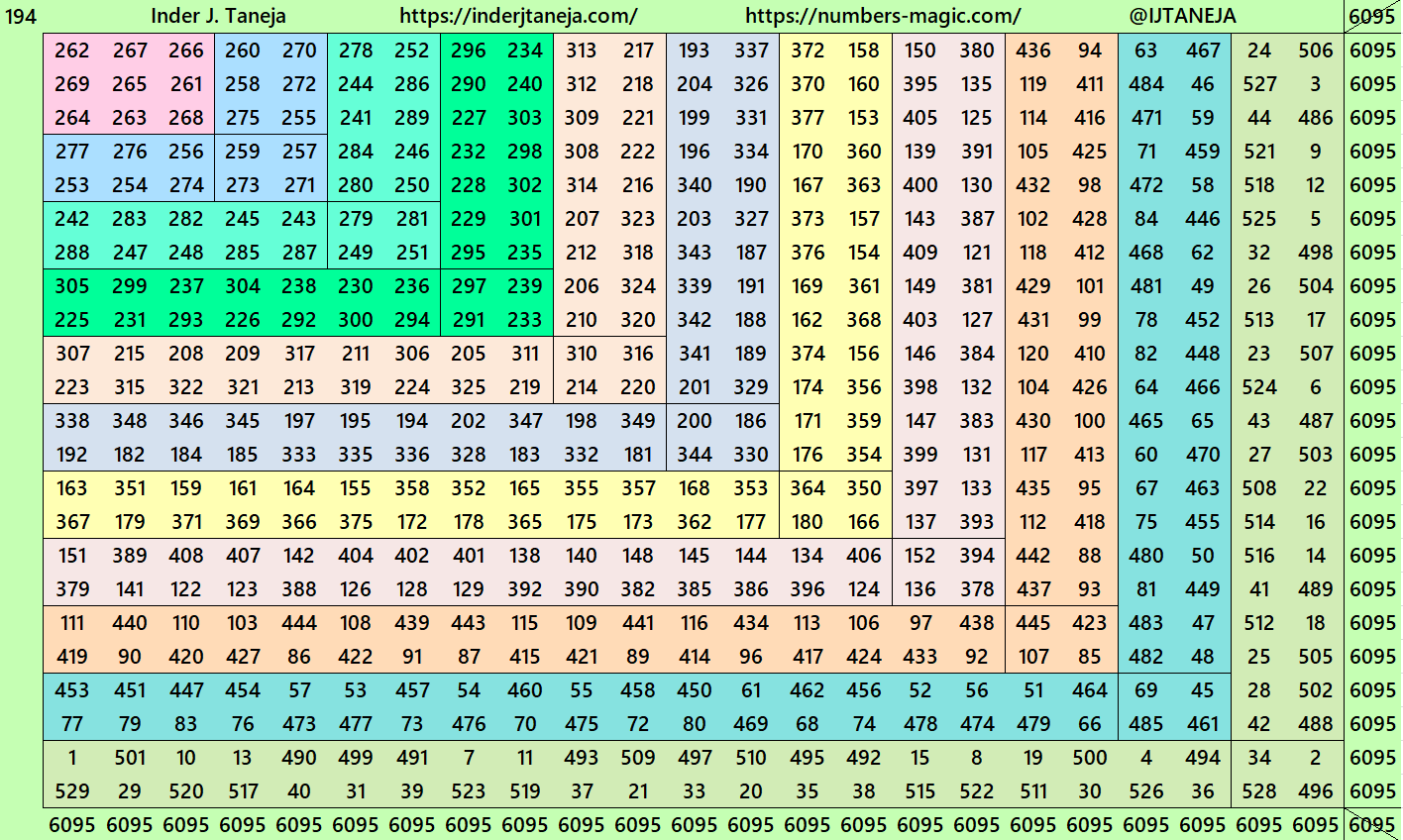
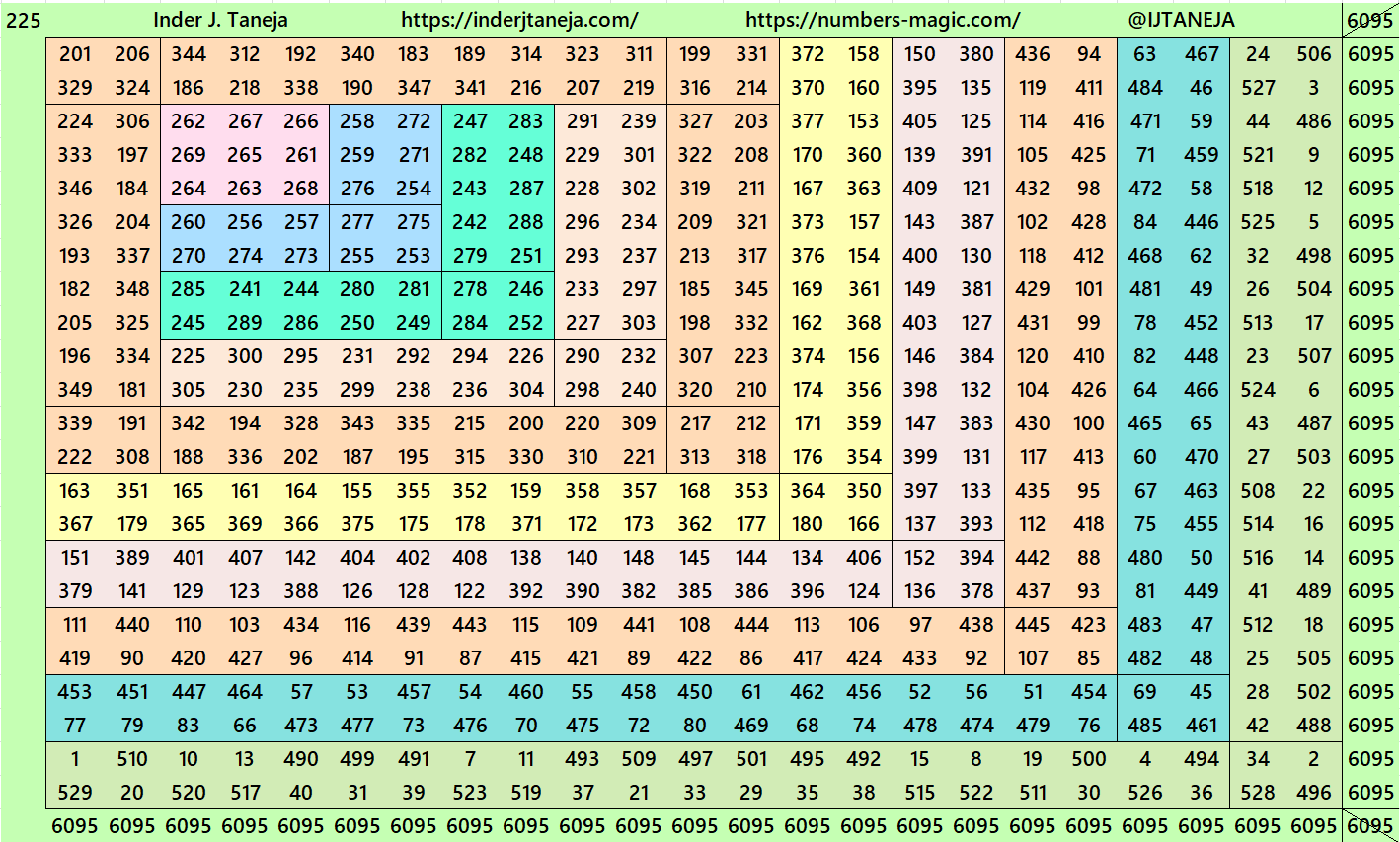
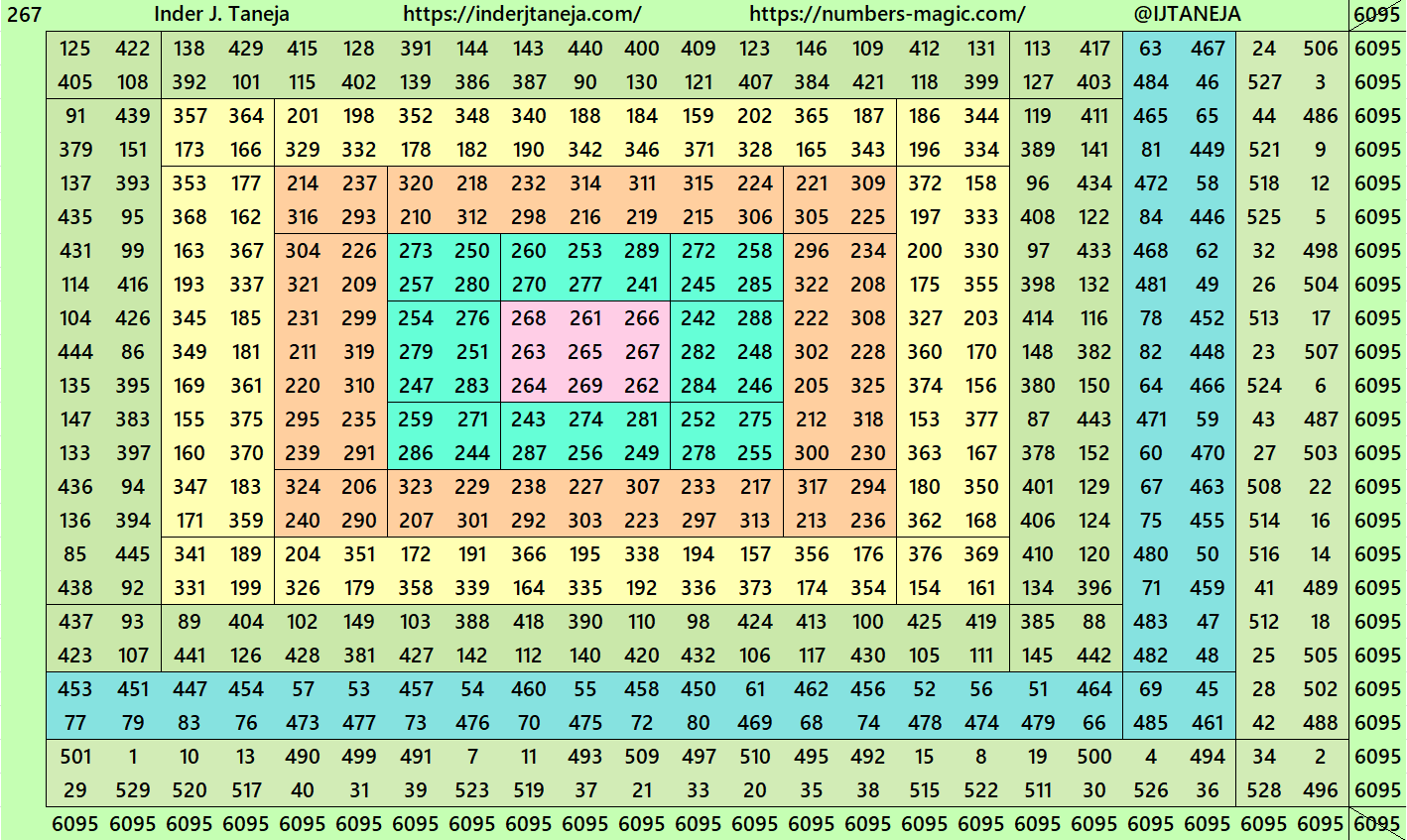

Cornered magic squares can be written in all the four directions. To do this, the reader can use the “rotate” script from the H. White’s site: Downloads (budshaw.ca). Also see the author’s work in all the four directions:
1. Inder J. Taneja, Cornered Magic Squares of Orders 5 to 10
2. Inder J. Taneja, Cornered Magic Squares of Orders 11 to 13
3. Inder J. Taneja, Cornered Magic Squares of Orders 14 to 24
Pdf file of Magic Squares of Order 23
There are total 370 magic squares of order 23. These are embedded as pdf file.