Bimagic Squares of Orders 256, 512 and 1024: Blocks of Order 16

Whole the work is done manually on excel sheets.

Below are bimagic squares written in blocks multiples of orders 16. This work brings magic squares multiples of 16 and 64, i.e., magic squares of orders 256, 512 and 1024. Most of the work is done manually by author in 2011. See the reference below:

Inder J. Taneja, Bimagic Squares of Bimagic Squares and an Open Problem, Febuarary 11, 2011, 2011, pp. 1-14, (22.02.2011), https://doi.org/10.48550/arXiv.1102.3052.

Before proceeding further below are the basic formulas to check the sums of magic and bimagic squares

• Magic Sum

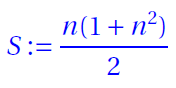
• Bimagic Sum

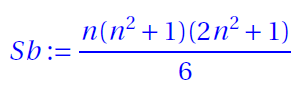
where n is the order the magic square.

Before giving the magic squares of orders 256, 512 and 1025, below are examples of bimagic squares of orders 16 and 64.

Bimagic Square of Order 16

In this case, we have

S_16×16 := 2056 and Sb_16×16 := 351576,

See below the bimagic square of order 16.

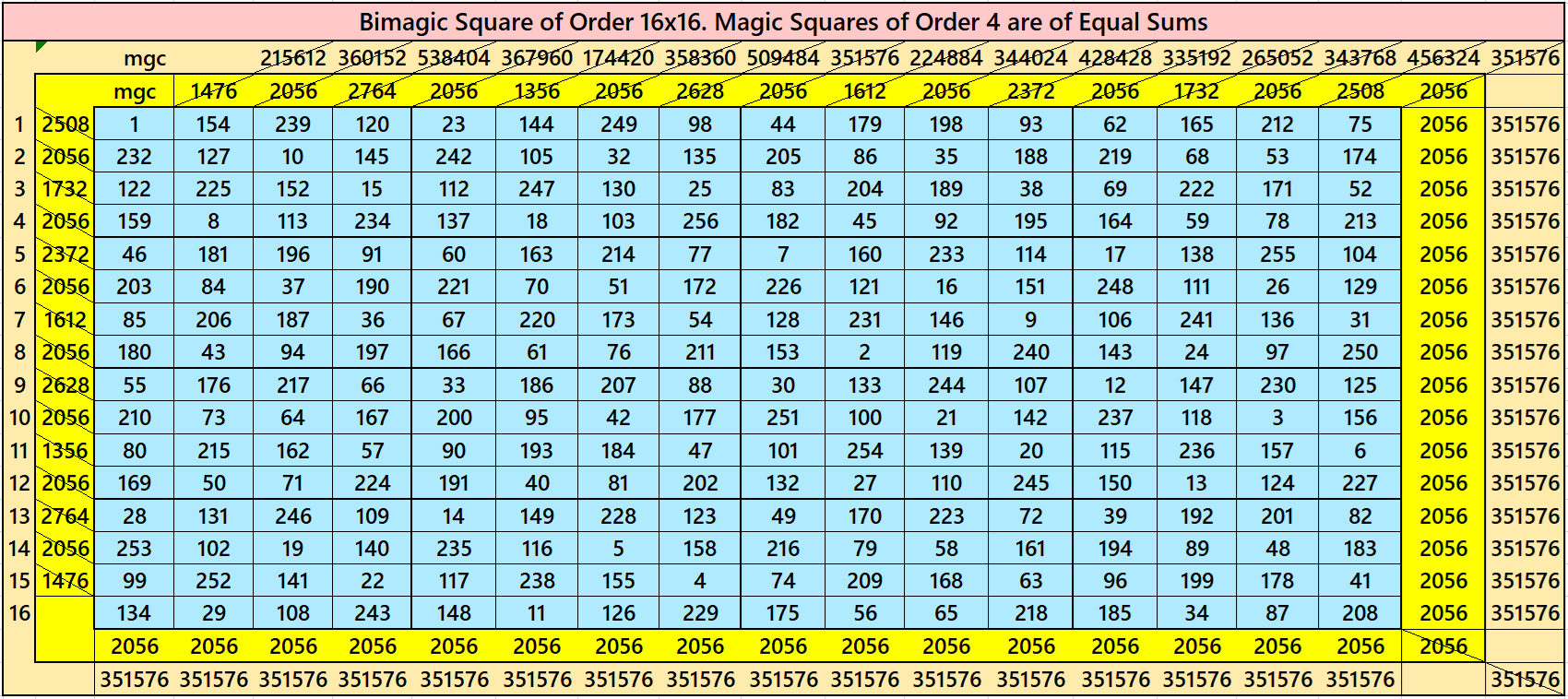
Magic squares of order 4 are of equal sums, S_4×4 :=(1/4) S_16×16=514.

Bimagic Square of Order 64: Blocks of Order 16

In this case, we have

S_64×64 :=131104 and Sb_64×64 := 358045024.

See below bimagic square of order 64 with blocks of order 16. These blocks of order 16 are bimagic squares with different bimagic sums, but the magic sums of all order 16 magic squares are same, i.e., S_16×16 := 32776. Moreover, magic sums of magic squares of order 4 are also same, i.e., S_4×4 := 8194

Bimagic Square of Order 256: Blocks of Order 16

Bimagic square of order 256×256 has the the following properties:

1. 4×4 are magic squares with equal magic sums, i.e., S_4×4:=131074.
2. 16×16 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_16×16:=524296.
3. 64×64 are bimagic squares with equal magic and bimagic sums, i.e., S_64×64:=2097184 and Sb_64×64:=91628066144.
4. 256×256 is a bimagic square with magic and bimagic sums, i.e., S_256×256:=8388736 and Sb_256×256:=366512264576.

Summarizing, we have a bimagic square of order 256×256, where blocks of orders 4×4, 16×16 and 64×64 are of equal magic sums. The magic squares of orders 16×16 bimagic with different bimagic sums. The magic squares of order 64×64 are of equal bimagic sums. These values are given in tables in an excel sheet attached with the work.

Bimagic Square of Order 512: Blocks of Order 16

Bimagic square of order 512×512 has the the following properties:

1. 4×4 are magic squares with equal magic sums, i.e., S_4×4 :=524290.
2. 16×16 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_16×16:=2097160.
3. 128×128 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_128×128:=16777280.
4. 512×512 is bimagic square with magic and bimagic sums, i.e., S_512×512:=67109120 and Sb_512×512:=11728191138560.

Summarizing, we have a bimagic square of order 512×512, where blocks of orders 4×4, 16×16, 128×128 are of equal magic sums. The magic squares of orders 16×16 and 128×128 are bimagic with different bimagic sums. These values are given in tables in an excel sheet attached with the work.

Bimagic Square of Order 1024: Blocks of Order 1024

It is bimagic square of order 1024×1024 with the following properties:

1. 4×4 are magic squares with equal magic sums, i.e., S_4×4 :=2097154.
2. 16×16 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_16×16:=8388616.
3. 64×64 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_64×64:=33554464.
4. 256×256 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_256×256:=134217856. Blocks of order 64×64 are with equal magic and bimagic sums.
5. 512×512 are bimagic squares with equal magic sums and different bimagic sums, i.e., S_512×512:=268435712.
6. 1024×1024 is bimagic squares with magic and bimagic sums, i.e., S_1024×1024:= 536871424 and Sb_1024×1024:=375300505818624.

Summarizing, we have a bimagic square of order 1024×1024, where blocks of orders 4×4, 16×16, 64×64 are and 128×128 are of equal magic sums. The magic squares of orders 16×16, 64×64 and 256×256 are bimagic with different bimagic sums. Each block of order 256×256, the bimagic sums of order 64×64 are the same. These values are given in tables in an excel sheet attached with the work.

References:

1. Block-Wise Construction of Bimagic Squares: Multiples of Orders 8 and 16.
2. Block-Wise Construction of Bimagic Squares Multiples of 25: Orders 25, 125 and 625.
3. Block-Wise Construction of Bimagic Squares Multiples of 9: Orders 9, 81 and 729.
4. Block-Wise Construction of Bimagic Squares of Orders 121 and 1331.