Few Examples of Magic Squares of Orders 20, 22 and 24 Using Bordered Magic Rectangles

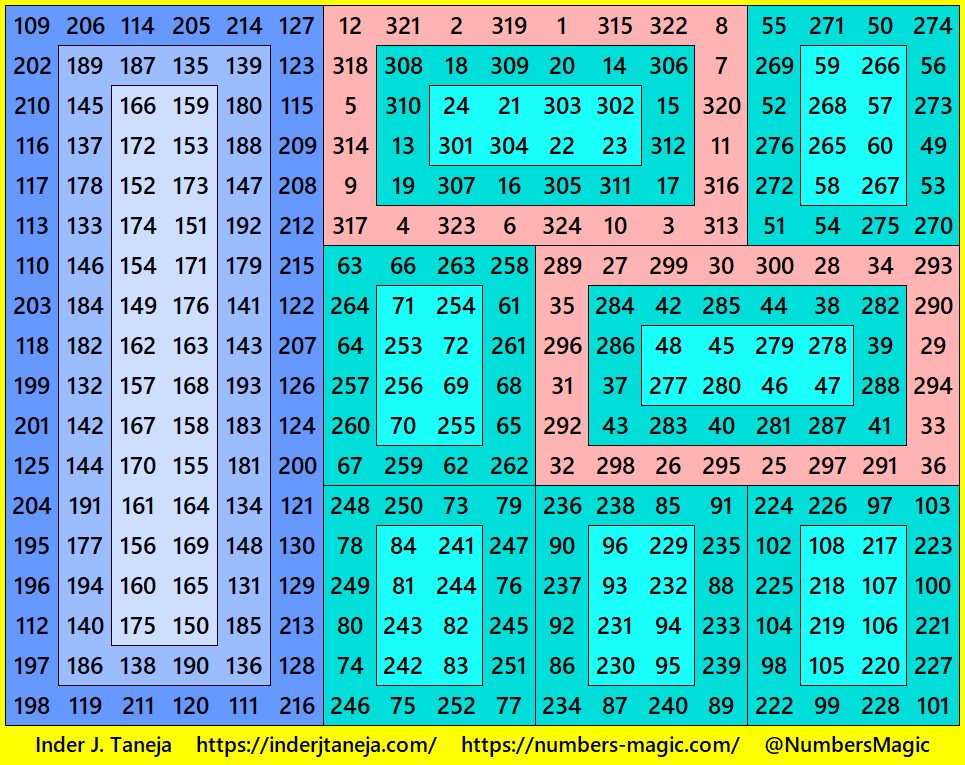
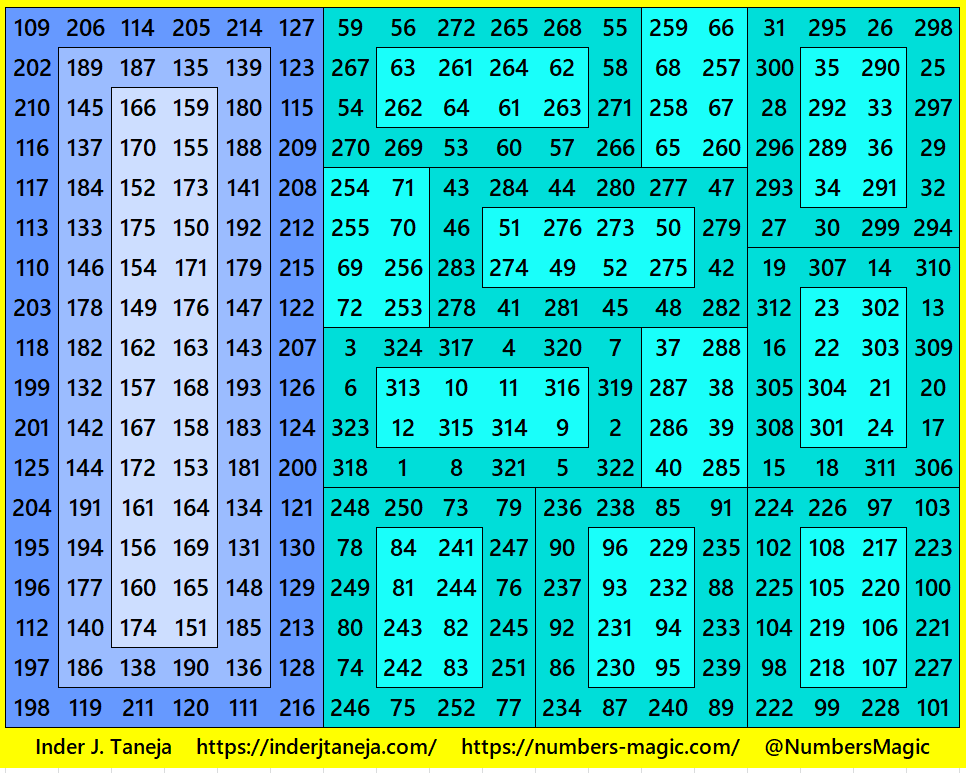
Recently, the author constructed even order magic squares using bordered magic rectangles. Below are the links to this work:

1. Inder J. Taneja, Different Styles of Magic Squares of Orders 8, 10 and 12 Using Bordered Magic Rectangles and the Formula (a+b)², Zenodo, September 18, 2022, pp. 1-30, https://doi.org/10.5281/zenodo.7090737.
   Also see the following links:
   a) Different Styles of Magic Squares of Orders 8 and 10 Using Bordered Magic Rectangles and the Formula (a+b)^2.
   b) Different Styles of Magic Squares of Order 12 Using Bordered Magic Rectangles and the Formula (a+b)^2.
2. Inder J. Taneja, Different Styles of Magic Squares of Order 14 Using Bordered Magic Rectangles and the Formula (a+b)², Zenodo, September 18, 2022, pp. 1-45, https://doi.org/10.5281/zenodo.7090764.
   Also see the following links:
   Different Styles of Magic Squares of Order 14 Using Bordered Magic Rectangles and the Formula (a+b)^2.
3. Inder J. Taneja, Different Styles of Magic Squares of Order 16 Using Bordered Magic Rectangles and the Formula (a+b)², Zenodo, September 18, 2022, pp. 1-85, https://doi.org/10.5281/zenodo.7090770.
   Also see the following links:
   Different Styles of Magic Squares of Order 16 Using Bordered Magic Rectangles and the Formula (a+b)^2
4. Inder J. Taneja, Different Styles of Magic Squares of Order 18 Using Bordered Magic Rectangles and the Formula (a+b)², Zenodo, September 23, 2022, pp. 1-136, https://doi.org/10.5281/zenodo.7108694.
   Also see the following links:
   Different Styles of Magic Squares of Order 18 Using Bordered Magic Rectangles and the Formula (a+b)^2.

Below are a few Examples of Magic Squares of Orders 20, 22 and 24.

• Part 1: Magic Squares of Order 20.
• Part 2: Magic Squares of Order 22.
• Part 3: Magic Squares of Order 24.

The examples given below can be downloaded at the following link:

• Inder J. Taneja, Few Examples of Magic Squares of Even Orders 20 to 30 Using Bordered Magic Rectangles, Zenodo, October 19, 2022, pp. 1-100, https://doi.org/10.5281/zenodo.7225886.

Part 1: Magic Squares of Order 20 With Bordered Magic Rectangles

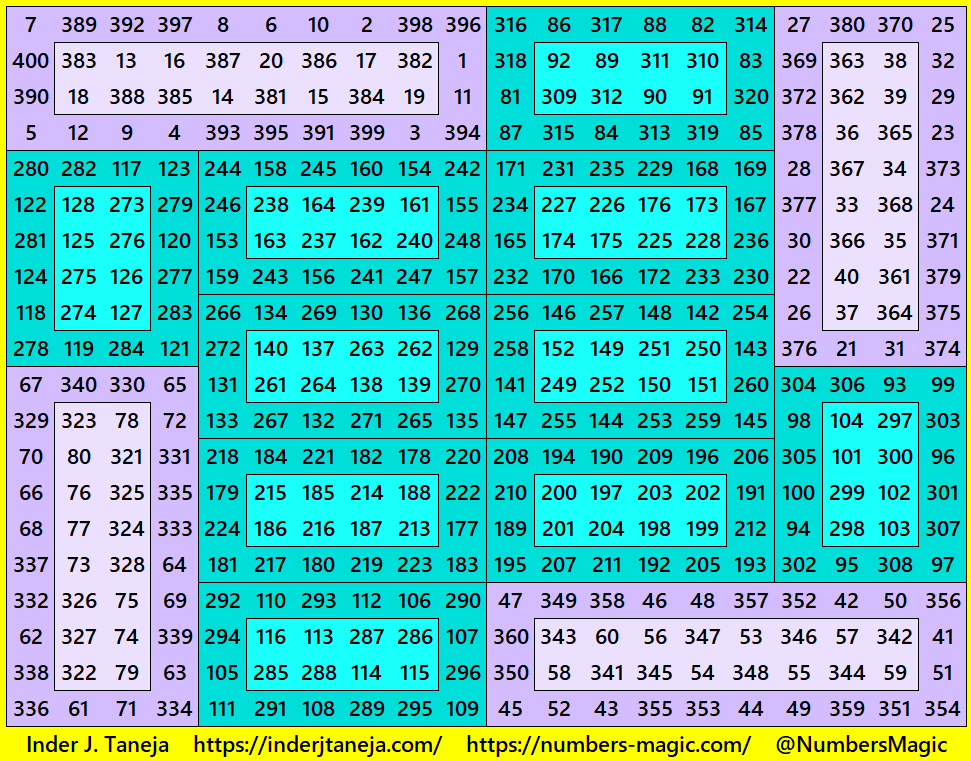
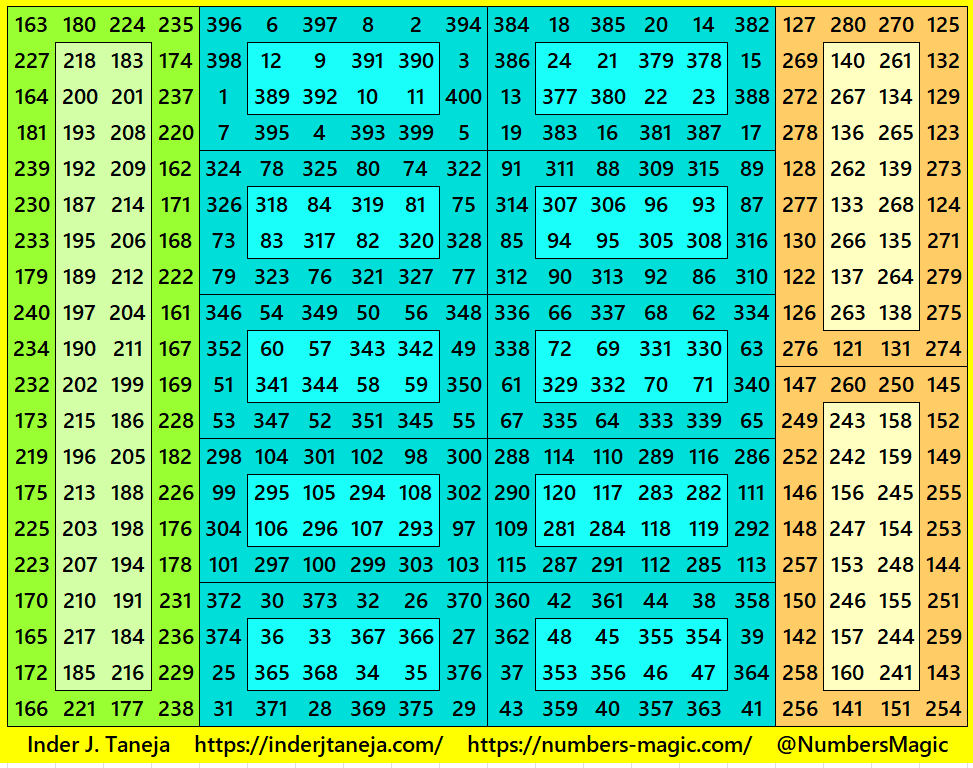
Part 2: Magic Squares of Order 22 With Bordered Magic Rectangles

Part 3: Magic Squares of Order 24 With Bordered Magic Rectangles